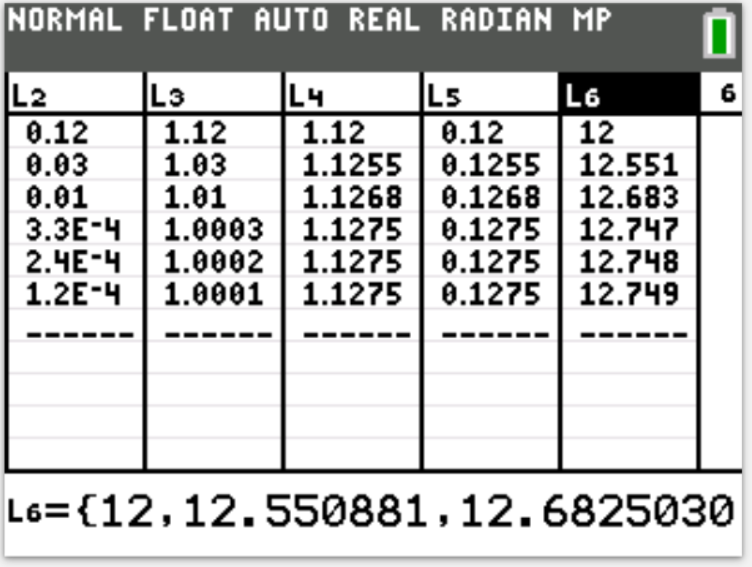
(9.) NSC Tshepo takes out a home loan over $20$ years to buy a house that costs $R1\:500\:000$.
$(9.1)$ Calculate the monthly installment if interest is charged at $10,5\%$ p.a, compounded monthly.
$(9.2)$ Calculate the outstanding balance immediately after the $144^{th}$ payment was made.
$ \underline{Amortization} \\[3ex] PV = R1500000 \\[3ex] t = 20\:years \\[3ex] r = 10.5\% = \dfrac{10.5}{100} = 0.105 \\[5ex] Compounded\:\:monthly \rightarrow m = 12 \\[3ex] PMT = ? \\[3ex] (9.1) \\[3ex] PMT = \dfrac{PV}{m} * \left[\dfrac{r}{1 - \left(1 + \dfrac{r}{m}\right)^{-mt}}\right] \\[10ex] PMT = \dfrac{1500000}{12} * \left[\dfrac{0.105}{1 - \left(1 + \dfrac{0.105}{12}\right)^{-1 * 12 * 20}}\right] \\[10ex] = 125000 * \left[\dfrac{0.105}{1 - \left(1 + 0.00875\right)^{-240}}\right] \\[7ex] = 125000 * \left[\dfrac{0.105}{1 - \left(1.00875\right)^{-240}}\right] \\[7ex] = 125000 * \left[\dfrac{0.105}{1 - 0.123580101}\right] \\[5ex] = 125000 * \left[\dfrac{0.105}{0.876419899}\right] \\[5ex] = \dfrac{125000 * 0.105}{0.876419899} \\[5ex] = \dfrac{13125}{0.876419899} \\[5ex] = 14975.6983 \\[3ex] \approx R14,975.70 \\[3ex] (9.2) \\[3ex] 12\:months = 1\:year \\[3ex] 144th\:\:payment \rightarrow \dfrac{144}{12} = 12th\:year\:\:payment \\[5ex] Remaining = 20 - 12 = 8\:years \\[3ex] $ Ask your students how they will calculate the outstanding balance after the $144^{th}$ payment.
Note their responses.
Some of the responses may include:
Find the total payment...for 20 years ...which is 240 months
Find the payment for 144 months
Subtract that payment from the total payment
...among other responses.
Remind them that these are Mortgage payments...Fixed Rate Mortgage
Show them how it works...using the example on my website
You can also use the Loan Amortization Calculator to confirm the calculations
Any monthly payment made is used to offset the interest earned during that period...first step
Then, any remaining balance is applied toward the principal.
The same principle applies to car payments and other amortized payments.
We can find the outstanding balance at the end of the $144th$ payemnt (at the end of $12$ years) in at least two ways.
Use any method you prefer.
First Method: Find the present value of the ordinary annuity for the remaining $8$ years.
OR
Second Method:
Compare to the previous response of "probably" one of your students.
But, explain the concept well for your students to understand.
Use the Compound Interest formula to calculate the future value of the loan amount for $12$ years.
Remember that the monthly payment of $14975.70$ was found using the Amortization formula for the entire term of the loan.
So, use the Future Value of an Ordinary Annuity formula and calculate the future value of that payment for $12$ years.
Then, subtract the future value of that payment from the future value of the loan amount.
$ \underline{First\:\:Method} \\[3ex] \underline{Present\:\:Value\:\:of\:\:Ordinary\:\:Annuity} \\[3ex] t = 8\:years \\[3ex] PMT = R14975.6983 \\[3ex] r = 0.105 \\[3ex] m = 12 \\[3ex] PV = m * PMT * \left[\dfrac{1 - \left(1 + \dfrac{r}{m}\right)^{-mt}}{r}\right] \\[10ex] PV = 12 * 14975.6983 * \left[\dfrac{1 - \left(1 + \dfrac{0.105}{12}\right)^{-1 * 12 * 8}}{0.105}\right] \\[10ex] = 179708.38 * \left[\dfrac{1 - \left(1 + 0.00875\right)^{-96}}{0.105}\right] \\[7ex] = 179708.38 * \left[\dfrac{1 - \left(1.00875\right)^{-96}}{0.105}\right] \\[7ex] = 179708.38 * \left[\dfrac{1 - 0.43329075}{0.105}\right] \\[5ex] = 179708.38 * \left[\dfrac{0.56670925}{0.105}\right] \\[5ex] = \dfrac{179708.38 * 0.56670925}{0.105} \\[5ex] = \dfrac{101842.401}{0.105} \\[5ex] = 969927.629 \\[3ex] \approx R969,927.63 \\[3ex] \underline{Second\:\:Method} \\[3ex] \underline{Compound\:\:Interest\:\:and\:\:Future\:\:Value\:\:of\:\:Ordinary\:\:Annuity} \\[3ex] \underline{Compound\:\:Interest} \\[3ex] P = R1500000 \\[3ex] r = 0.105 \\[3ex] t = 12\:years \\[3ex] m = 12 \\[3ex] A = P\left(1 + \dfrac{r}{m}\right)^{mt} \\[7ex] A = 1500000\left(1 + \dfrac{0.105}{12}\right)^{12 * 12} \\[7ex] = 1500000\left(1 + 0.00875\right)^{144} \\[7ex] = 1500000\left(1.00875\right)^{144} \\[7ex] = 1500000(3.50615308) \\[3ex] = R5259229.62 \\[3ex] \underline{Future\:\:Value\:\:of\:\:Ordinary\:\:Annuity} \\[3ex] m = 12 \\[3ex] PMT = R14975.6983 \\[3ex] r = 0.105 \\[3ex] t = 12\:years \\[3ex] FV = m * PMT * \left[\dfrac{\left(1 + \dfrac{r}{m}\right)^{mt} - 1}{r}\right] \\[10ex] FV = 12 * 14975.6983 * \left[\dfrac{\left(1 + \dfrac{0.105}{12}\right)^{12 * 12} - 1}{0.105}\right] \\[10ex] = 179708.38 * \left[\dfrac{\left(1 + 0.00875\right)^{144} - 1}{0.105}\right] \\[7ex] = 179708.38 * \left[\dfrac{\left(1.00875\right)^{144} - 1}{0.105}\right] \\[7ex] = 179708.38 * \left[\dfrac{3.50615308 - 1}{0.105}\right] \\[5ex] = 179708.38 * \left[\dfrac{2.50615308}{0.105}\right] \\[5ex] = \dfrac{179708.38 * 2.50615308}{0.105} \\[5ex] = \dfrac{450376.71}{0.105} \\[5ex] = R4289302 \\[3ex] \underline{Balance\:\:after\:\:144th\:\:payment} \\[3ex] Balance = 5259229.62 - 4289302 = R969,927.62 \\[3ex] $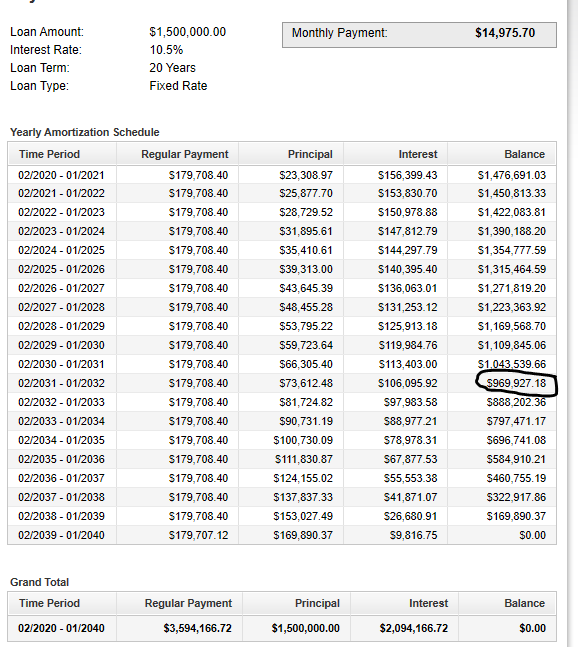
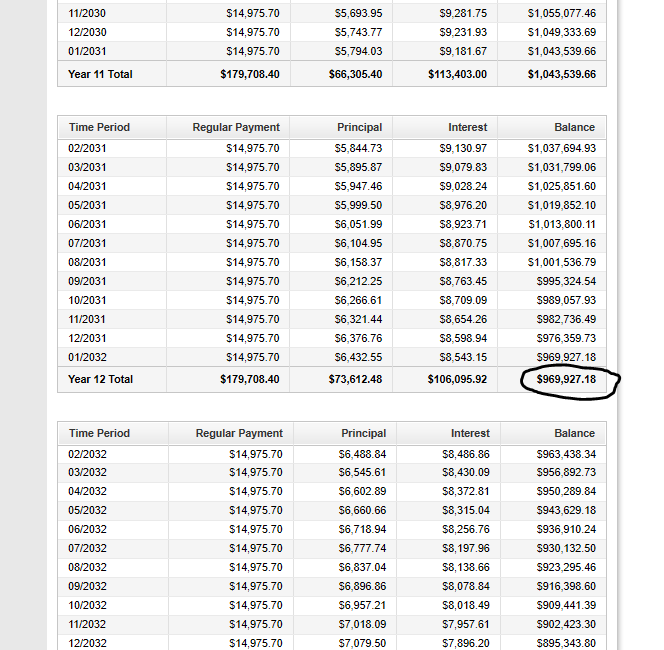
$(9.1)$ Calculate the monthly installment if interest is charged at $10,5\%$ p.a, compounded monthly.
$(9.2)$ Calculate the outstanding balance immediately after the $144^{th}$ payment was made.
$ \underline{Amortization} \\[3ex] PV = R1500000 \\[3ex] t = 20\:years \\[3ex] r = 10.5\% = \dfrac{10.5}{100} = 0.105 \\[5ex] Compounded\:\:monthly \rightarrow m = 12 \\[3ex] PMT = ? \\[3ex] (9.1) \\[3ex] PMT = \dfrac{PV}{m} * \left[\dfrac{r}{1 - \left(1 + \dfrac{r}{m}\right)^{-mt}}\right] \\[10ex] PMT = \dfrac{1500000}{12} * \left[\dfrac{0.105}{1 - \left(1 + \dfrac{0.105}{12}\right)^{-1 * 12 * 20}}\right] \\[10ex] = 125000 * \left[\dfrac{0.105}{1 - \left(1 + 0.00875\right)^{-240}}\right] \\[7ex] = 125000 * \left[\dfrac{0.105}{1 - \left(1.00875\right)^{-240}}\right] \\[7ex] = 125000 * \left[\dfrac{0.105}{1 - 0.123580101}\right] \\[5ex] = 125000 * \left[\dfrac{0.105}{0.876419899}\right] \\[5ex] = \dfrac{125000 * 0.105}{0.876419899} \\[5ex] = \dfrac{13125}{0.876419899} \\[5ex] = 14975.6983 \\[3ex] \approx R14,975.70 \\[3ex] (9.2) \\[3ex] 12\:months = 1\:year \\[3ex] 144th\:\:payment \rightarrow \dfrac{144}{12} = 12th\:year\:\:payment \\[5ex] Remaining = 20 - 12 = 8\:years \\[3ex] $ Ask your students how they will calculate the outstanding balance after the $144^{th}$ payment.
Note their responses.
Some of the responses may include:
Find the total payment...for 20 years ...which is 240 months
Find the payment for 144 months
Subtract that payment from the total payment
...among other responses.
Remind them that these are Mortgage payments...Fixed Rate Mortgage
Show them how it works...using the example on my website
You can also use the Loan Amortization Calculator to confirm the calculations
Any monthly payment made is used to offset the interest earned during that period...first step
Then, any remaining balance is applied toward the principal.
The same principle applies to car payments and other amortized payments.
We can find the outstanding balance at the end of the $144th$ payemnt (at the end of $12$ years) in at least two ways.
Use any method you prefer.
First Method: Find the present value of the ordinary annuity for the remaining $8$ years.
OR
Second Method:
Compare to the previous response of "probably" one of your students.
But, explain the concept well for your students to understand.
Use the Compound Interest formula to calculate the future value of the loan amount for $12$ years.
Remember that the monthly payment of $14975.70$ was found using the Amortization formula for the entire term of the loan.
So, use the Future Value of an Ordinary Annuity formula and calculate the future value of that payment for $12$ years.
Then, subtract the future value of that payment from the future value of the loan amount.
$ \underline{First\:\:Method} \\[3ex] \underline{Present\:\:Value\:\:of\:\:Ordinary\:\:Annuity} \\[3ex] t = 8\:years \\[3ex] PMT = R14975.6983 \\[3ex] r = 0.105 \\[3ex] m = 12 \\[3ex] PV = m * PMT * \left[\dfrac{1 - \left(1 + \dfrac{r}{m}\right)^{-mt}}{r}\right] \\[10ex] PV = 12 * 14975.6983 * \left[\dfrac{1 - \left(1 + \dfrac{0.105}{12}\right)^{-1 * 12 * 8}}{0.105}\right] \\[10ex] = 179708.38 * \left[\dfrac{1 - \left(1 + 0.00875\right)^{-96}}{0.105}\right] \\[7ex] = 179708.38 * \left[\dfrac{1 - \left(1.00875\right)^{-96}}{0.105}\right] \\[7ex] = 179708.38 * \left[\dfrac{1 - 0.43329075}{0.105}\right] \\[5ex] = 179708.38 * \left[\dfrac{0.56670925}{0.105}\right] \\[5ex] = \dfrac{179708.38 * 0.56670925}{0.105} \\[5ex] = \dfrac{101842.401}{0.105} \\[5ex] = 969927.629 \\[3ex] \approx R969,927.63 \\[3ex] \underline{Second\:\:Method} \\[3ex] \underline{Compound\:\:Interest\:\:and\:\:Future\:\:Value\:\:of\:\:Ordinary\:\:Annuity} \\[3ex] \underline{Compound\:\:Interest} \\[3ex] P = R1500000 \\[3ex] r = 0.105 \\[3ex] t = 12\:years \\[3ex] m = 12 \\[3ex] A = P\left(1 + \dfrac{r}{m}\right)^{mt} \\[7ex] A = 1500000\left(1 + \dfrac{0.105}{12}\right)^{12 * 12} \\[7ex] = 1500000\left(1 + 0.00875\right)^{144} \\[7ex] = 1500000\left(1.00875\right)^{144} \\[7ex] = 1500000(3.50615308) \\[3ex] = R5259229.62 \\[3ex] \underline{Future\:\:Value\:\:of\:\:Ordinary\:\:Annuity} \\[3ex] m = 12 \\[3ex] PMT = R14975.6983 \\[3ex] r = 0.105 \\[3ex] t = 12\:years \\[3ex] FV = m * PMT * \left[\dfrac{\left(1 + \dfrac{r}{m}\right)^{mt} - 1}{r}\right] \\[10ex] FV = 12 * 14975.6983 * \left[\dfrac{\left(1 + \dfrac{0.105}{12}\right)^{12 * 12} - 1}{0.105}\right] \\[10ex] = 179708.38 * \left[\dfrac{\left(1 + 0.00875\right)^{144} - 1}{0.105}\right] \\[7ex] = 179708.38 * \left[\dfrac{\left(1.00875\right)^{144} - 1}{0.105}\right] \\[7ex] = 179708.38 * \left[\dfrac{3.50615308 - 1}{0.105}\right] \\[5ex] = 179708.38 * \left[\dfrac{2.50615308}{0.105}\right] \\[5ex] = \dfrac{179708.38 * 2.50615308}{0.105} \\[5ex] = \dfrac{450376.71}{0.105} \\[5ex] = R4289302 \\[3ex] \underline{Balance\:\:after\:\:144th\:\:payment} \\[3ex] Balance = 5259229.62 - 4289302 = R969,927.62 \\[3ex] $


(10.) HSC Mathematics Standard 1 James invests $5000 per annum simple interest for 2
years, while Sally invests
$5000 at 3% per annum compounded annually for 2 years.
For the given investments, which of the following statements is TRUE?
A. James earns more interest than Sally.
B. Sally earns more interest than James.
C. James and Sally earn the same amount of interest.
D. There is not enough information to compare the interest earned by James and Sally.
For one year, James and Sally will earn the same interest.
However, for two years?
Well, it's evident Sally will earn more interest because it is compounded annually for anything time beyond one year.
Let us show it.
$ \underline{James:\;\;Simple\;\;Interest} \\[3ex] P = \$5000 \\[3ex] r = 3\% = \dfrac{3}{100} = 0.03 \\[5ex] t = 2\;years \\[3ex] SI = Prt \\[3ex] = 5000(0.03)(2) \\[3ex] = 300 \\[3ex] = \$300.00 \\[3ex] \underline{Sally:\;\;Compound\;\;Interest} \\[3ex] compounded\;\;annually \\[3ex] P = \$5000 \\[3ex] r = 3\% = \dfrac{3}{100} = 0.03 \\[5ex] t = 2\;years \\[3ex] m = 1 \\[3ex] CI = P\left(1 + \dfrac{r}{m}\right)^{mt} - P \\[5ex] = 5000 * \left(1 + \dfrac{0.03}{1}\right)^{1 * 2} - 5000 \\[5ex] = 5000(1 + 0.03)^2 - 5000 \\[3ex] = 5000(1.03)^2 - 5000 \\[3ex] = 5000(1.0609) - 5000 \\[3ex] = 5304.5 - 5000 \\[3ex] = 304.5 \\[3ex] \approx \$304.50 \\[3ex] $ Because $\$304.50$ is greater than $\$300.00$, Sally earns more interest than James.
Student: Mr. C, you mentioned earlier that if the time was 1 year, then the simple interest would be equal to the compound interest.
Teacher: If the compound interest was compounded annually...yes
And if the principal and interest rate was the same for both the simple interest and the compound interest
Student: Okay, can you give an example?
Teacher: With numbers, or without numbers, or both?
Student: Both...
Teacher: Let's assume a time of 1 year for the question, rather than 2 years
$ P = \$5000 \\[3ex] r = 0.03 \\[3ex] t = 1\;year \\[3ex] \underline{Simple\;\;Interest} \\[3ex] SI = Prt \\[3ex] = 5000(0.03)(1) \\[3ex] = 150 \\[3ex] = \$150.00 \\[5ex] \underline{Compound\;\;Interest} \\[3ex] compounded\;\;annually \implies m = 1 \\[3ex] A = P\left(1 + \dfrac{r}{m}\right)^{mt} \\[5ex] = 5000 * \left(1 + \dfrac{0.03}{1}\right)^{1 * 1} \\[5ex] = 5000 * (1 + 0.03)^1 \\[3ex] = 5000(1.03) \\[3ex] = 5150 \\[3ex] CI = A - P \\[3ex] = 5150 - 5000 \\[3ex] = 150 \\[3ex] = \$150.00 \\[3ex] $ Both interests (simple interest and compound interest) are the same.
Here is the example of doing the calculations without numbers
For the given investments, which of the following statements is TRUE?
A. James earns more interest than Sally.
B. Sally earns more interest than James.
C. James and Sally earn the same amount of interest.
D. There is not enough information to compare the interest earned by James and Sally.
For one year, James and Sally will earn the same interest.
However, for two years?
Well, it's evident Sally will earn more interest because it is compounded annually for anything time beyond one year.
Let us show it.
$ \underline{James:\;\;Simple\;\;Interest} \\[3ex] P = \$5000 \\[3ex] r = 3\% = \dfrac{3}{100} = 0.03 \\[5ex] t = 2\;years \\[3ex] SI = Prt \\[3ex] = 5000(0.03)(2) \\[3ex] = 300 \\[3ex] = \$300.00 \\[3ex] \underline{Sally:\;\;Compound\;\;Interest} \\[3ex] compounded\;\;annually \\[3ex] P = \$5000 \\[3ex] r = 3\% = \dfrac{3}{100} = 0.03 \\[5ex] t = 2\;years \\[3ex] m = 1 \\[3ex] CI = P\left(1 + \dfrac{r}{m}\right)^{mt} - P \\[5ex] = 5000 * \left(1 + \dfrac{0.03}{1}\right)^{1 * 2} - 5000 \\[5ex] = 5000(1 + 0.03)^2 - 5000 \\[3ex] = 5000(1.03)^2 - 5000 \\[3ex] = 5000(1.0609) - 5000 \\[3ex] = 5304.5 - 5000 \\[3ex] = 304.5 \\[3ex] \approx \$304.50 \\[3ex] $ Because $\$304.50$ is greater than $\$300.00$, Sally earns more interest than James.
Student: Mr. C, you mentioned earlier that if the time was 1 year, then the simple interest would be equal to the compound interest.
Teacher: If the compound interest was compounded annually...yes
And if the principal and interest rate was the same for both the simple interest and the compound interest
Student: Okay, can you give an example?
Teacher: With numbers, or without numbers, or both?
Student: Both...
Teacher: Let's assume a time of 1 year for the question, rather than 2 years
$ P = \$5000 \\[3ex] r = 0.03 \\[3ex] t = 1\;year \\[3ex] \underline{Simple\;\;Interest} \\[3ex] SI = Prt \\[3ex] = 5000(0.03)(1) \\[3ex] = 150 \\[3ex] = \$150.00 \\[5ex] \underline{Compound\;\;Interest} \\[3ex] compounded\;\;annually \implies m = 1 \\[3ex] A = P\left(1 + \dfrac{r}{m}\right)^{mt} \\[5ex] = 5000 * \left(1 + \dfrac{0.03}{1}\right)^{1 * 1} \\[5ex] = 5000 * (1 + 0.03)^1 \\[3ex] = 5000(1.03) \\[3ex] = 5150 \\[3ex] CI = A - P \\[3ex] = 5150 - 5000 \\[3ex] = 150 \\[3ex] = \$150.00 \\[3ex] $ Both interests (simple interest and compound interest) are the same.
Here is the example of doing the calculations without numbers