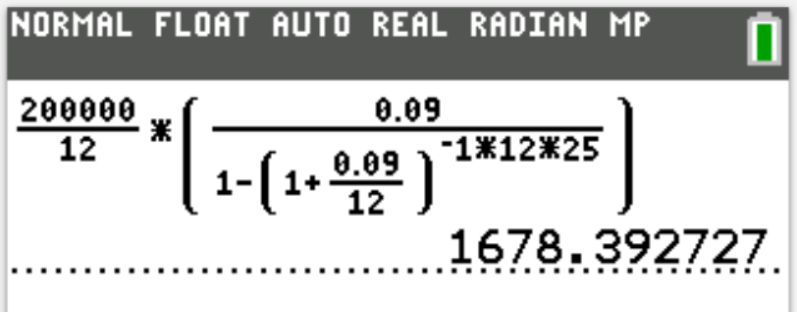
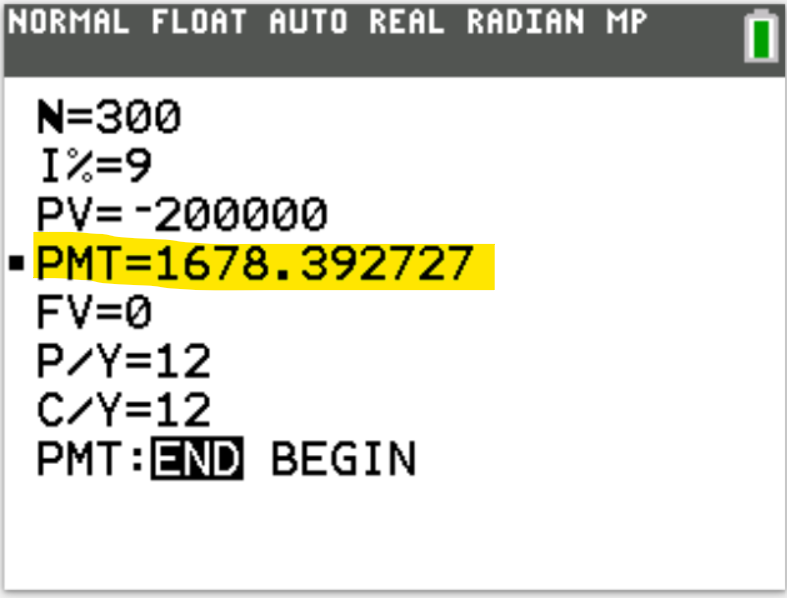
(7.) The Luke's family just closed on a 15-year mortgage home for $120,000 at 7% per annum.
Angel Bank is financing the home.
Prepare a Loan Amortization Schedule for the mortgage.
This is a case of Amortization
$ PV = \$120000 \\[3ex] t = 15\: years \\[3ex] r = 7\% = \dfrac{7}{100} = 0.07 \\[5ex] Compounded\:\:monthly \rightarrow m = 12 \\[3ex] PMT = ? \\[3ex] PMT = \dfrac{PV}{m} * \left[\dfrac{r}{1 - \left(1 + \dfrac{r}{m}\right)^{-mt}}\right] \\[10ex] PMT = \dfrac{PV}{m} * \left[\dfrac{r}{1 - \left(1 + \dfrac{r}{m}\right)^{-1 * m * t}}\right] \\[10ex] PMT = \dfrac{120000}{12} * \left[\dfrac{0.07}{1 - \left(1 + \dfrac{0.07}{12}\right)^{-1 * 12 * 15}}\right] \\[10ex] = 10000 * \left[\dfrac{0.07}{1 - \left(1 + 0.00583333333\right)^{-180}}\right] \\[7ex] = 10000 * \left[\dfrac{0.07}{1 - \left(1.00583333333\right)^{-180}}\right] \\[7ex] = 10000 * \left[\dfrac{0.07}{1 - 0.351006914}\right] \\[5ex] = 10000 * \left[\dfrac{0.07}{0.648993086}\right] \\[5ex] = \dfrac{10000 * 0.07}{0.648993086} \\[5ex] = \dfrac{700}{0.648993086} \\[5ex] = 1078.59393 \\[3ex] PMT \approx \$1078.59\\[3ex] Number\:\:of\:\:payments = m * t \\[3ex] Number\:\:of\:\:payments = 12 * 15 = 180\:payments \\[3ex] $
$ \underline{Payment\:\:Number\:1} \\[3ex] Interest\:\:Payment = P * r * t = 120000 * 0.07 * \dfrac{1}{12} = \$700.00 \\[5ex] Principal\:\:Payment = Monthly\:\:Payment - Interest\:\:Payment \\[3ex] Principal\:\:Payment = 1078.59 - 700.00 = \$378.59 \\[3ex] Balance\:\:of\:\:Loan = Principal\:\:Balance - Principal\:\:Payment \\[3ex] Balance\:\:of\:\:Loan = 120000.00 - 378.59 = \$119621.41 \\[3ex] $ NOTE: For the first payment:
The Principal Balance is the Amount of Mortgage
The Principal is also the Amount of Mortgage.
For the second payment:
The Principal Balance is the Principal for the second payment.
The Principal Balance is also the Balance of Loan after the first payment.
For the third payment:
The Principal Balance is the Principal for the third payment.
The Principal Balance is also the Balance of Loan after the second payment.
...and so on...and so forth...
$ \underline{Payment\:\:Number\:2} \\[3ex] Interest\:\:Payment = P * r * t = 119621.41 * 0.07 * \dfrac{1}{12} = \$697.79 \\[5ex] Principal\:\:Payment = Monthly\:\:Payment - Interest\:\:Payment \\[3ex] Principal\:\:Payment = 1078.59 - 697.79 = \$380.80 \\[3ex] Balance\:\:of\:\:Loan = Principal\:\:Balance - Principal\:\:Payment \\[3ex] Balance\:\:of\:\:Loan = 119621.41 - 380.80 = \$119240.61 \\[3ex] $ Complete the rest of the Loan Amortization Table.
Check your answers with the calculator (please see the top page)
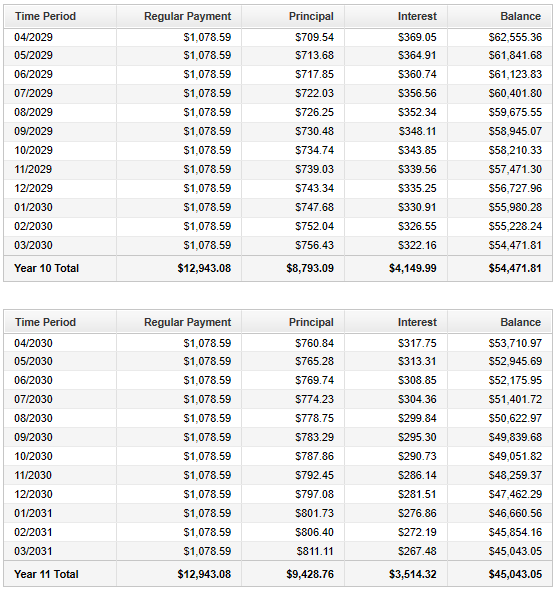
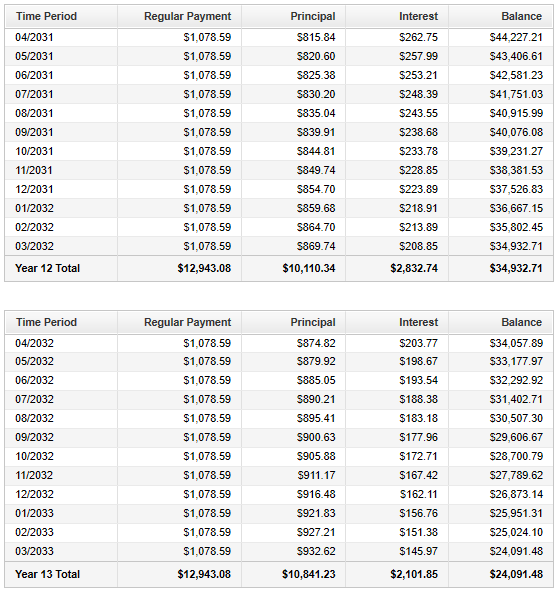
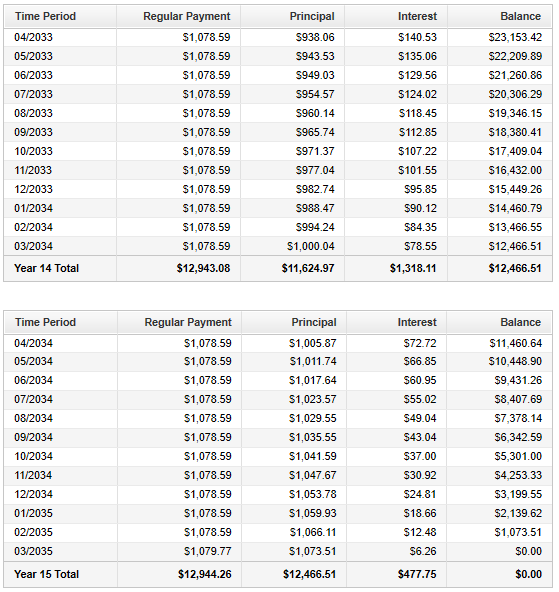
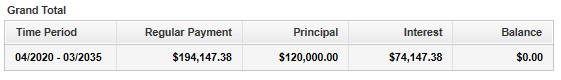
You can also check your answers using the table below.



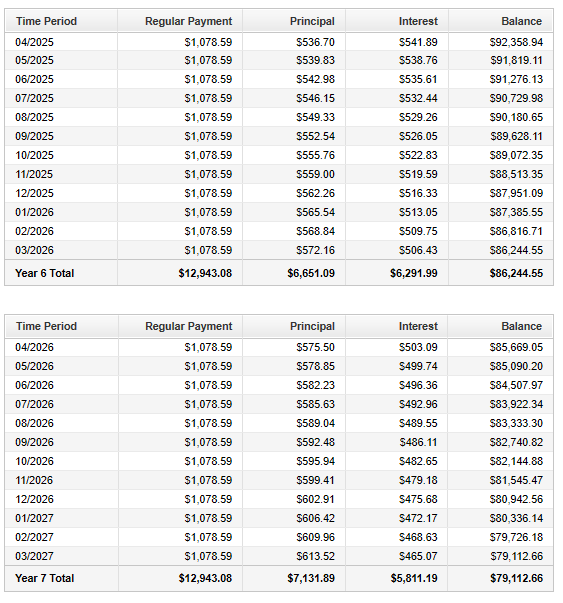
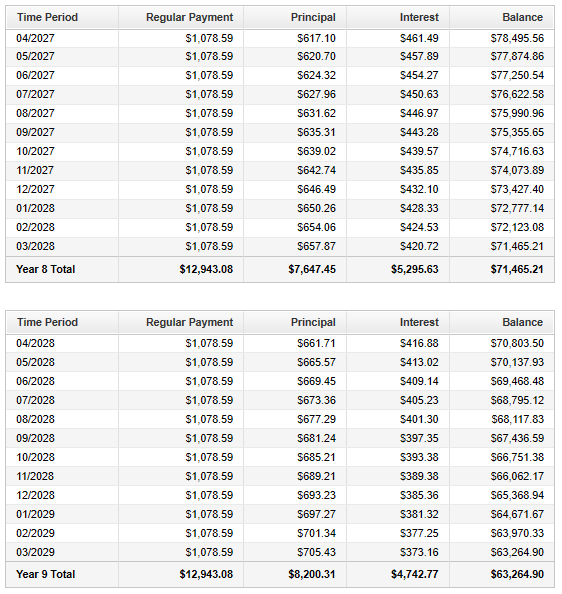
Angel Bank is financing the home.
Prepare a Loan Amortization Schedule for the mortgage.
This is a case of Amortization
$ PV = \$120000 \\[3ex] t = 15\: years \\[3ex] r = 7\% = \dfrac{7}{100} = 0.07 \\[5ex] Compounded\:\:monthly \rightarrow m = 12 \\[3ex] PMT = ? \\[3ex] PMT = \dfrac{PV}{m} * \left[\dfrac{r}{1 - \left(1 + \dfrac{r}{m}\right)^{-mt}}\right] \\[10ex] PMT = \dfrac{PV}{m} * \left[\dfrac{r}{1 - \left(1 + \dfrac{r}{m}\right)^{-1 * m * t}}\right] \\[10ex] PMT = \dfrac{120000}{12} * \left[\dfrac{0.07}{1 - \left(1 + \dfrac{0.07}{12}\right)^{-1 * 12 * 15}}\right] \\[10ex] = 10000 * \left[\dfrac{0.07}{1 - \left(1 + 0.00583333333\right)^{-180}}\right] \\[7ex] = 10000 * \left[\dfrac{0.07}{1 - \left(1.00583333333\right)^{-180}}\right] \\[7ex] = 10000 * \left[\dfrac{0.07}{1 - 0.351006914}\right] \\[5ex] = 10000 * \left[\dfrac{0.07}{0.648993086}\right] \\[5ex] = \dfrac{10000 * 0.07}{0.648993086} \\[5ex] = \dfrac{700}{0.648993086} \\[5ex] = 1078.59393 \\[3ex] PMT \approx \$1078.59\\[3ex] Number\:\:of\:\:payments = m * t \\[3ex] Number\:\:of\:\:payments = 12 * 15 = 180\:payments \\[3ex] $
| Loan Amortization Schedule for the Luke's Family | |||
|---|---|---|---|
|
Annual $\%$Rate: $7\%$ Amount of Mortgage: $\$120,000$ Number of Monthly Payments: $180$ |
Monthly Payment: $\$1,078.59$ Term: Years $15$, Months $0$ |
||
| Payment Number | Interest Payment | Principal Payment | Balance of Loan |
| $1$ | $\$700.00$ | $\$378.59$ | $\$119,621.41$ |
| $2$ | $\$697.79$ | $\$380.80$ | $\$119,240.61$ |
| Complete it | Complete it | Complete it | Complete it |
$ \underline{Payment\:\:Number\:1} \\[3ex] Interest\:\:Payment = P * r * t = 120000 * 0.07 * \dfrac{1}{12} = \$700.00 \\[5ex] Principal\:\:Payment = Monthly\:\:Payment - Interest\:\:Payment \\[3ex] Principal\:\:Payment = 1078.59 - 700.00 = \$378.59 \\[3ex] Balance\:\:of\:\:Loan = Principal\:\:Balance - Principal\:\:Payment \\[3ex] Balance\:\:of\:\:Loan = 120000.00 - 378.59 = \$119621.41 \\[3ex] $ NOTE: For the first payment:
The Principal Balance is the Amount of Mortgage
The Principal is also the Amount of Mortgage.
For the second payment:
The Principal Balance is the Principal for the second payment.
The Principal Balance is also the Balance of Loan after the first payment.
For the third payment:
The Principal Balance is the Principal for the third payment.
The Principal Balance is also the Balance of Loan after the second payment.
...and so on...and so forth...
$ \underline{Payment\:\:Number\:2} \\[3ex] Interest\:\:Payment = P * r * t = 119621.41 * 0.07 * \dfrac{1}{12} = \$697.79 \\[5ex] Principal\:\:Payment = Monthly\:\:Payment - Interest\:\:Payment \\[3ex] Principal\:\:Payment = 1078.59 - 697.79 = \$380.80 \\[3ex] Balance\:\:of\:\:Loan = Principal\:\:Balance - Principal\:\:Payment \\[3ex] Balance\:\:of\:\:Loan = 119621.41 - 380.80 = \$119240.61 \\[3ex] $ Complete the rest of the Loan Amortization Table.
Check your answers with the calculator (please see the top page)
You can also check your answers using the table below.









(8.) Payments are made at the end of each month into an ordinary annuity earning interest at the rate of
2.25% per year compounded monthly for $9\dfrac{1}{4}$ years.
If the present value of the annuity is $45,000, determine the size of each payment.
This is a case of Amortization
$ PV = \$45000 \\[3ex] t = 9\dfrac{1}{4} = 9.25\: years \\[5ex] r = 2.25\% = \dfrac{2.25}{100} = 0.0225 \\[5ex] Compounded\:\:monthly \rightarrow m = 12 \\[3ex] PMT = ? \\[3ex] PMT = \dfrac{PV}{m} * \left[\dfrac{r}{1 - \left(1 + \dfrac{r}{m}\right)^{-mt}}\right] \\[10ex] PMT = \dfrac{PV}{m} * \left[\dfrac{r}{1 - \left(1 + \dfrac{r}{m}\right)^{-1 * m * t}}\right] \\[10ex] PMT = \dfrac{45000}{12} * \left[\dfrac{0.0225}{1 - \left(1 + \dfrac{0.0225}{12}\right)^{-1 * 12 * 9.25}}\right] \\[10ex] = 3750 * \left[\dfrac{0.0225}{1 - \left(1 + 0.001875\right)^{-111}}\right] \\[7ex] = 3750 * \left[\dfrac{0.0225}{1 - \left(1.001875\right)^{-111}}\right] \\[7ex] = 3750 * \left[\dfrac{0.0225}{1 - 0.8122637906}\right] \\[5ex] = 3750 * \left[\dfrac{0.0225}{0.1877362094}\right] \\[5ex] = \dfrac{3750 * 0.0225}{0.1877362094} \\[5ex] = \dfrac{84.375}{0.1877362094} \\[5ex] = 449.4338107 \\[3ex] PMT \approx \$449.43 $
If the present value of the annuity is $45,000, determine the size of each payment.
This is a case of Amortization
$ PV = \$45000 \\[3ex] t = 9\dfrac{1}{4} = 9.25\: years \\[5ex] r = 2.25\% = \dfrac{2.25}{100} = 0.0225 \\[5ex] Compounded\:\:monthly \rightarrow m = 12 \\[3ex] PMT = ? \\[3ex] PMT = \dfrac{PV}{m} * \left[\dfrac{r}{1 - \left(1 + \dfrac{r}{m}\right)^{-mt}}\right] \\[10ex] PMT = \dfrac{PV}{m} * \left[\dfrac{r}{1 - \left(1 + \dfrac{r}{m}\right)^{-1 * m * t}}\right] \\[10ex] PMT = \dfrac{45000}{12} * \left[\dfrac{0.0225}{1 - \left(1 + \dfrac{0.0225}{12}\right)^{-1 * 12 * 9.25}}\right] \\[10ex] = 3750 * \left[\dfrac{0.0225}{1 - \left(1 + 0.001875\right)^{-111}}\right] \\[7ex] = 3750 * \left[\dfrac{0.0225}{1 - \left(1.001875\right)^{-111}}\right] \\[7ex] = 3750 * \left[\dfrac{0.0225}{1 - 0.8122637906}\right] \\[5ex] = 3750 * \left[\dfrac{0.0225}{0.1877362094}\right] \\[5ex] = \dfrac{3750 * 0.0225}{0.1877362094} \\[5ex] = \dfrac{84.375}{0.1877362094} \\[5ex] = 449.4338107 \\[3ex] PMT \approx \$449.43 $